import numpy as np
import matplotlib.pyplot as plt
plt.style.use('fivethirtyeight')
Introduction to Engineering Dynamics#
Newtonian Mechanics#
There are three laws that define Newtonian Mechanics:
An object at rest will stay at rest and an object in motion will remain in motion unless acted upon by an external force \(\sum\mathbf{F}=\mathbf{0}\) and \(\sum\mathbf{M} = \mathbf{0}\)
The change in momentum of a body is proportional to the force applied, \(\sum \mathbf{F} = \frac{d}{dt}\left(m\mathbf{v}\right)\) and \(\sum\mathbf{M} = \frac{d}{dt}\left(\mathbf{h}\right)\)
For every applied force, there is an equal and opposite reaction force
import sympy
sympy.var('m, g, P_x, P_y, R_y, W_P, L, x')
Fx = P_x
Fy = P_y + R_y - W_P - m*g
Mz = -L/2*m*g + L*R_y - x*W_P
eqns = sympy.Matrix([Fx, Fy, Mz])
A = sympy.solve((eqns[0], eqns[1], eqns[2]), (P_x, P_y, R_y))
Py_function = sympy.lambdify((x, L, W_P, m, g), A[P_y], 'numpy')
Ry_function = sympy.lambdify((x, L, W_P, m, g), A[R_y], 'numpy')
Bridge example: live load no acceleration#
Consider a 50-kg person travelling from the left-to-right edges of the bridge at a constant velocity. The acceleration of the person is 0 and the bridge has 0 acceleration.
\(v_{person} = 10~\frac{m}{s}\)
\(x_{person} = \int_0^tv_{person}dt = 10t~m\)
The first law defines the study of static structures and objects that are moving slowly or have negligible momentum, \(m\mathbf{v}\). In order to keep the bridge from moving, the total applied force \(\mathbf{F}\) and moments \(\mathbf{M}\) must be equal to \(\mathbf{0}\).
\(\left[\begin{matrix} \sum F_x\\ \sum F_y\\ \sum M_z \end{matrix}\right] = \left[\begin{matrix} P_{x}\\ P_{y} + R_{y} - W_{P} - g m\\ L R_{y} - \frac{L g m}{2} - W_{P} x \end{matrix}\right] = \left[\begin{matrix} 0\\ 0\\ 0\\ \end{matrix}\right]\)
Note: If you set up the equations by separating the unknown variables, \([P_x,~P_y,~R_y]\), from the known variables, you can set up a linear algebra problem. For more information, check out the excellent video series 3Blue 1Brown - Essence of Linear Algebra
\(\left[\begin{matrix} ~1 & 0 & 0\\ 0 & 1 & 1 \\ 0 & 0 &L \end{matrix}\right] \left[\begin{array} ~P_x\\ P_y\\ R_y\end{array}\right]= \left[\begin{matrix} 0\\ W_{P} + mg \\ \frac{mgL}{2}+W_Px\\ \end{matrix}\right]\)
Solving for the reaction forces, you have three equations,
\(P_x = 0\)
\(P_y = W_P +mg - \frac{mg}{2}-W_P\frac{x}{L}\)
\(R_y = \frac{mg}{2}+W_P\frac{x}{L}\)
g = 9.81
L = 10
m = 100
Wp = 50*g
Px = lambda x: 0*x
Py = lambda x: Wp + m*g/2 - Wp*x/L
Ry = lambda x: m*g/2 +Wp*x/L
t = np.linspace(0, 1)
v = 10
x = v*t
plt.plot(x, Px(x), label = r'$P_x$')
plt.plot(x, Py(x), label = r'$P_y$')
plt.plot(x, Ry(x), label = r'$R_y$')
plt.legend()
plt.xlabel('walker position (m)')
plt.ylabel('reaction force (N)')
Text(0, 0.5, 'reaction force (N)')
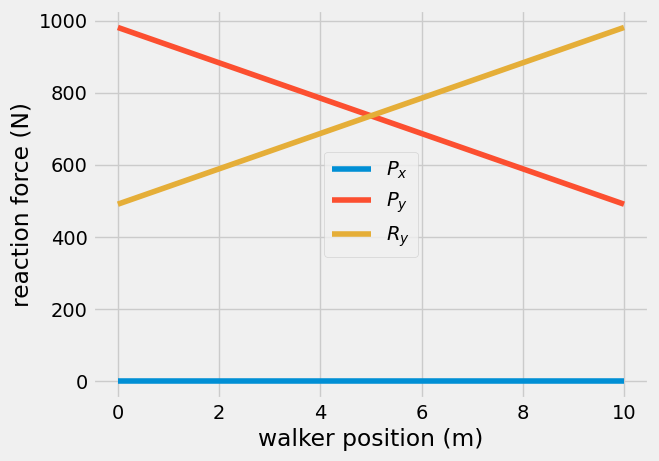
Here you can see the reaction forces at each end of the bridge as a function of the person moving from left, \(x=0~m\) to right, \(x=10~m\). The reaction force increases as the walker moves away from the support.
You can watch the reaction forces change in the animation below.
from matplotlib import animation
from IPython.display import HTML
fig, ax = plt.subplots(1,1);
ax.plot(x, x*0, '-', linewidth = 10, label = 'bridge')
Q = ax.quiver([0, 10],
[0, 0],
[0, 0],
[Py(x[1]), Ry(x[1])],
scale = 3500, label = 'reaction forces')
mark, = ax.plot(x[1], 0.01, 'v', markersize = 30, label = 'walker position')
ax.legend(loc = 'lower center')
ax.set_xlim(-0.5, 10.5)
ax.set_ylim(-0.1, 0.1)
def animate(i):
"""updates the horizontal and vertical vector components by a
fixed increment on each frame
"""
Q.set_UVC(np.array([0, 0]),
np.array([Py(x[i]), Ry(x[i])]))
mark.set_data(x[i], 0.05)
return Q, mark
# you need to set blit=False, or the first set of arrows never gets
# cleared on subsequent frames
anim = animation.FuncAnimation(fig, animate,
frames = range(len(t)-1), blit=False)
HTML(anim.to_html5_video())
---------------------------------------------------------------------------
RuntimeError Traceback (most recent call last)
Cell In[5], line 31
27 # you need to set blit=False, or the first set of arrows never gets
28 # cleared on subsequent frames
29 anim = animation.FuncAnimation(fig, animate,
30 frames = range(len(t)-1), blit=False)
---> 31 HTML(anim.to_html5_video())
File /opt/hostedtoolcache/Python/3.9.23/x64/lib/python3.9/site-packages/matplotlib/animation.py:1265, in Animation.to_html5_video(self, embed_limit)
1262 path = Path(tmpdir, "temp.m4v")
1263 # We create a writer manually so that we can get the
1264 # appropriate size for the tag
-> 1265 Writer = writers[mpl.rcParams['animation.writer']]
1266 writer = Writer(codec='h264',
1267 bitrate=mpl.rcParams['animation.bitrate'],
1268 fps=1000. / self._interval)
1269 self.save(str(path), writer=writer)
File /opt/hostedtoolcache/Python/3.9.23/x64/lib/python3.9/site-packages/matplotlib/animation.py:128, in MovieWriterRegistry.__getitem__(self, name)
126 if self.is_available(name):
127 return self._registered[name]
--> 128 raise RuntimeError(f"Requested MovieWriter ({name}) not available")
RuntimeError: Requested MovieWriter (ffmpeg) not available
Error in callback <function _draw_all_if_interactive at 0x7f358cf48c10> (for post_execute), with arguments args (),kwargs {}:
---------------------------------------------------------------------------
RuntimeError Traceback (most recent call last)
File /opt/hostedtoolcache/Python/3.9.23/x64/lib/python3.9/site-packages/matplotlib/pyplot.py:268, in _draw_all_if_interactive()
266 def _draw_all_if_interactive() -> None:
267 if matplotlib.is_interactive():
--> 268 draw_all()
File /opt/hostedtoolcache/Python/3.9.23/x64/lib/python3.9/site-packages/matplotlib/_pylab_helpers.py:131, in Gcf.draw_all(cls, force)
129 for manager in cls.get_all_fig_managers():
130 if force or manager.canvas.figure.stale:
--> 131 manager.canvas.draw_idle()
File /opt/hostedtoolcache/Python/3.9.23/x64/lib/python3.9/site-packages/matplotlib/backend_bases.py:1905, in FigureCanvasBase.draw_idle(self, *args, **kwargs)
1903 if not self._is_idle_drawing:
1904 with self._idle_draw_cntx():
-> 1905 self.draw(*args, **kwargs)
File /opt/hostedtoolcache/Python/3.9.23/x64/lib/python3.9/site-packages/matplotlib/backends/backend_agg.py:387, in FigureCanvasAgg.draw(self)
384 # Acquire a lock on the shared font cache.
385 with (self.toolbar._wait_cursor_for_draw_cm() if self.toolbar
386 else nullcontext()):
--> 387 self.figure.draw(self.renderer)
388 # A GUI class may be need to update a window using this draw, so
389 # don't forget to call the superclass.
390 super().draw()
File /opt/hostedtoolcache/Python/3.9.23/x64/lib/python3.9/site-packages/matplotlib/artist.py:95, in _finalize_rasterization.<locals>.draw_wrapper(artist, renderer, *args, **kwargs)
93 @wraps(draw)
94 def draw_wrapper(artist, renderer, *args, **kwargs):
---> 95 result = draw(artist, renderer, *args, **kwargs)
96 if renderer._rasterizing:
97 renderer.stop_rasterizing()
File /opt/hostedtoolcache/Python/3.9.23/x64/lib/python3.9/site-packages/matplotlib/artist.py:72, in allow_rasterization.<locals>.draw_wrapper(artist, renderer)
69 if artist.get_agg_filter() is not None:
70 renderer.start_filter()
---> 72 return draw(artist, renderer)
73 finally:
74 if artist.get_agg_filter() is not None:
File /opt/hostedtoolcache/Python/3.9.23/x64/lib/python3.9/site-packages/matplotlib/figure.py:3169, in Figure.draw(self, renderer)
3166 finally:
3167 self.stale = False
-> 3169 DrawEvent("draw_event", self.canvas, renderer)._process()
File /opt/hostedtoolcache/Python/3.9.23/x64/lib/python3.9/site-packages/matplotlib/backend_bases.py:1217, in Event._process(self)
1215 def _process(self):
1216 """Process this event on ``self.canvas``, then unset ``guiEvent``."""
-> 1217 self.canvas.callbacks.process(self.name, self)
1218 self._guiEvent_deleted = True
File /opt/hostedtoolcache/Python/3.9.23/x64/lib/python3.9/site-packages/matplotlib/cbook.py:303, in CallbackRegistry.process(self, s, *args, **kwargs)
301 except Exception as exc:
302 if self.exception_handler is not None:
--> 303 self.exception_handler(exc)
304 else:
305 raise
File /opt/hostedtoolcache/Python/3.9.23/x64/lib/python3.9/site-packages/matplotlib/cbook.py:87, in _exception_printer(exc)
85 def _exception_printer(exc):
86 if _get_running_interactive_framework() in ["headless", None]:
---> 87 raise exc
88 else:
89 traceback.print_exc()
File /opt/hostedtoolcache/Python/3.9.23/x64/lib/python3.9/site-packages/matplotlib/cbook.py:298, in CallbackRegistry.process(self, s, *args, **kwargs)
296 if func is not None:
297 try:
--> 298 func(*args, **kwargs)
299 # this does not capture KeyboardInterrupt, SystemExit,
300 # and GeneratorExit
301 except Exception as exc:
File /opt/hostedtoolcache/Python/3.9.23/x64/lib/python3.9/site-packages/matplotlib/animation.py:892, in Animation._start(self, *args)
889 self._fig.canvas.mpl_disconnect(self._first_draw_id)
891 # Now do any initial draw
--> 892 self._init_draw()
894 # Add our callback for stepping the animation and
895 # actually start the event_source.
896 self.event_source.add_callback(self._step)
File /opt/hostedtoolcache/Python/3.9.23/x64/lib/python3.9/site-packages/matplotlib/animation.py:1733, in FuncAnimation._init_draw(self)
1725 warnings.warn(
1726 "Can not start iterating the frames for the initial draw. "
1727 "This can be caused by passing in a 0 length sequence "
(...)
1730 "it may be exhausted due to a previous display or save."
1731 )
1732 return
-> 1733 self._draw_frame(frame_data)
1734 else:
1735 self._drawn_artists = self._init_func()
File /opt/hostedtoolcache/Python/3.9.23/x64/lib/python3.9/site-packages/matplotlib/animation.py:1752, in FuncAnimation._draw_frame(self, framedata)
1748 self._save_seq = self._save_seq[-self._save_count:]
1750 # Call the func with framedata and args. If blitting is desired,
1751 # func needs to return a sequence of any artists that were modified.
-> 1752 self._drawn_artists = self._func(framedata, *self._args)
1754 if self._blit:
1756 err = RuntimeError('The animation function must return a sequence '
1757 'of Artist objects.')
Cell In[5], line 23, in animate(i)
17 """updates the horizontal and vertical vector components by a
18 fixed increment on each frame
19 """
20 Q.set_UVC(np.array([0, 0]),
21 np.array([Py(x[i]), Ry(x[i])]))
---> 23 mark.set_data(x[i], 0.05)
25 return Q, mark
File /opt/hostedtoolcache/Python/3.9.23/x64/lib/python3.9/site-packages/matplotlib/lines.py:665, in Line2D.set_data(self, *args)
662 else:
663 x, y = args
--> 665 self.set_xdata(x)
666 self.set_ydata(y)
File /opt/hostedtoolcache/Python/3.9.23/x64/lib/python3.9/site-packages/matplotlib/lines.py:1289, in Line2D.set_xdata(self, x)
1276 """
1277 Set the data array for x.
1278
(...)
1286 set_ydata
1287 """
1288 if not np.iterable(x):
-> 1289 raise RuntimeError('x must be a sequence')
1290 self._xorig = copy.copy(x)
1291 self._invalidx = True
RuntimeError: x must be a sequence
An example with \(a\neq 0\)#
Acceleration of a falling ball \(a\neq 0\)#
In the previous bridge example, the person and bridge had 0 acceleration. Consider an falling object. Suppose you want to use video capture of a falling ball to compute the acceleration of gravity.
Watch the video of the ball falling.
from IPython.display import YouTubeVideo
vid = YouTubeVideo("xQ4znShlK5A")
display(vid)
We learn from the video that the marks on the panel are every \(0.25\rm{m}\), and on the website they say that the strobe light flashes at about 15 Hz (that’s 15 times per second). The final image on Flickr notes that the strobe fired 16.8 times per second.
The ball is freefalling, so the acceleration is constant, \(a = g = 9.81~\frac{m}{s^2}\). Its not moving left or right, so you can describe its position and velocity as
\(x(t) = x_0\)
\(y(t) = y_0 + \dot{y}_0t +\frac{g}{2}t^2\)
where the initial position is \((x_0,~y_0)\), the initial velocity is \((0,~\dot{y}_0)\), and \(t\) is elapsed time. Take a look at the final frame of the compiled positions below.
reader = imageio.get_reader('https://go.gwu.edu/engcomp3vidmit', format='mp4')
image = reader.get_data(1100)
plt.imshow(image, interpolation='nearest');

The first position after releasing the ball, is just below the first white line at 125 pixels. The average distance between markers is 495 pixels/meter, so the camera would measure an acceleration
\(g = 9.81~\frac{m}{s^2}\cdot\frac{495~pixels}{1~meter}=4850~\frac{pixels}{s^2}\).
With a little guess-and-check, the initial velocity just below the first white marker is \(\dot{y}_0=800~\frac{pixels}{s}\). The position on the image is then,
\(y(t) = 125 + 800 t + \frac{4850}{2}t^2~pixels\).
The frames are plotted on top of the image below.
x0 = 720
y0 = 125
bars = np.array([115, 980])
scale = np.diff(bars)/1.75
print(scale, 'px/m')
g = 9.81*scale # m/s^2*d*0.25 px/m = px/s^2
t = np.arange(0, 8, 1)*1/16.8
x = np.ones(len(t))*x0
y = y0 + 800*t + g*t**2/2
plt.clf()
plt.imshow(image)
plt.scatter(x, y, s = 80, facecolor='none', edgecolors='b')
[494.28571429] px/m
<matplotlib.collections.PathCollection at 0x7f993dbd3bb0>

Wrapping up#
In this notebook, you looked at two situations
forces and motion when acceleration is 0
motion when acceleration is \(a\neq0\)
Next, you will define properties of kinetics (forces, energy, and impulse) and kinematics (motion described by position, velocity, acceleration).